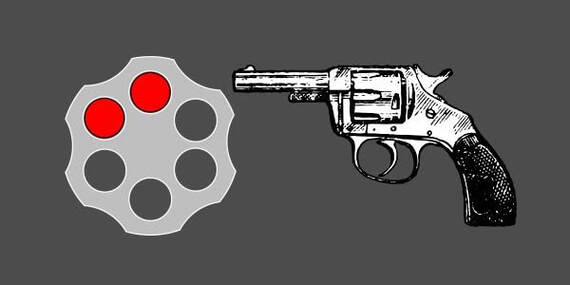
There is a six chamber gun with two bullets next to each other. The trigger is pulled and it doesn't fire. What are the odds of it firing and being killed if the trigger is pulled again? [I slightly edited the question and shortened a lengthy mathematical analysis of the revolver by Jerry Liu on Quora, a Software Engineering Intern at Google 2020-present. Hopefully, I kept what is essential. SFPD officers now use a pistol, not a revolver. The edited version follows.]
When the trigger is pulled, chambers rotate, the chamber right beside the current one is moved into the trigger position, and the gun will continuously cycle between the 6 chambers.
Now … if we’re considering the chance of dying, or a bullet firing after the trigger is pulled twice … in words, this means that the gun is triggered. If it fires, it doesn’t need to be triggered again. If it doesn’t fire, then it will be triggered a second time. As most agree, the first trigger has a 1/3 or 33.33% chance of firing. We then need to figure in the percentage of the second trigger firing if the first trigger doesn’t fire.
The key is that this means one of the bullets cannot be fired without the other being fired first. The short answer is that the odds of it firing on the second trigger pull is 25%. [Jerry gave more explanation, but I left this part out.]
The chance of the first trigger not firing is 2/3 or 66.66%. Now that we have this percentage, we can calculate the percentage the same way as my original approach, obtaining 1/4 or 25%. [see above]
Since these events are tied in sequence, their probabilities need to be multiplied together or 1/4 x 2/3, which is equal to 1/6. This is then added to the 1/3 chance from the first trigger. 1/3 + 1/6 = 2/6 + 1/6, giving a final answer of 3/6 or a 50% chance of being killed. Wow, it is 50-50...anyway.
Altan (13) is learning about probabilities, permutations, and combinations. Should I give him this problem? Many people in my neck of the woods own a gun or rifle.
RSS Feed